Rational Exponents
Definition of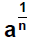 
If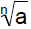 
a represents a real
number and n≥2 is an integer, then
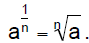
If n is odd and
• a is positive, then
is positive.
• a is negative, then
is negative.
• a is zero, then
is zero.
If n is even and
• a is positive, then
is positive.
• a is negative, then
is not a real number
• a is zero, then
is also zero.
Example 1: Use radical notation to rewrite each
expression.
Simplify, if possible.
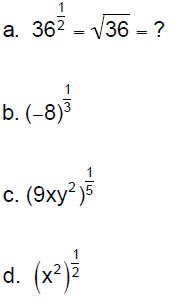
Example 2: Rewrite each expression using rational exponents.
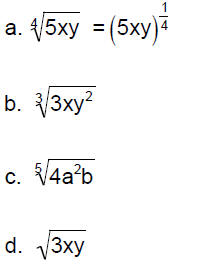
Definition of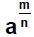 
If
represents a real
number and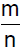
is a positive rational number,
n≥2, then
Note that if n is even and a is negative,does
not represent a real
number and 
is not a real number.
Example 3: Use radical notation to rewrite each of the
following
and then simplify.
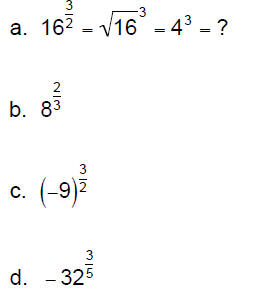
Example 4: Rewrite with rational exponents.
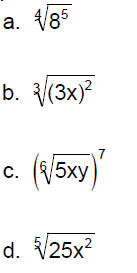
Definition of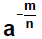 
If is a nonzero real
number, then
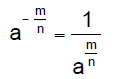
Example 5: Rewrite each of the following with a positive
exponent. Simplify, if possible. Assume all variables represent
nonnegative quantities.
Properties of Rational Exponents
If m and n are rational exponents, and a and b are real
numbers for
which the following expressions are defined, then
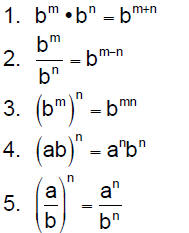
Example 6: Simplify the following expressions with
rational
exponents. Express all answers with positive exponents.
Assume all variables represent nonnegative quantities.
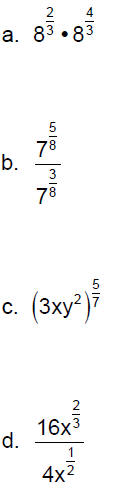
Simplifying Radical Expressions Using Rational Exponents
To simplify a radical expression by using rational
exponents:
1. Rewrite each radical expression as an exponential
expression with a rational exponent.
2. Simplify using properties of rational exponents.
3. Rewrite your answer in radical notation when rational
exponents still appear.
Example 7: Use rational exponents to simplify. Assume all
variables represent nonnegative quantities.
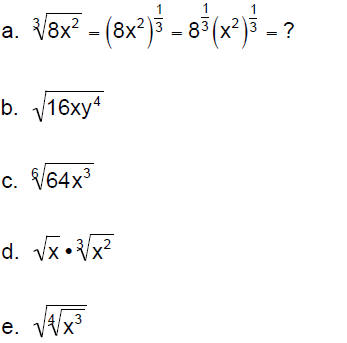
Application of Rational Exponents
Example 8: The function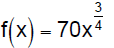
models the number of
calories per day, f(x), that a person needs to maintain life in
terms of that person’s weight, x, in kilograms. (1 kilogram is
approximately 2.2 pounds.) Use the model and a calculator to
find how many calories per day are required to maintain life for
a person who weighs 55 kilograms (about 121 pounds). Round
your answer to the nearest calorie.
Example 9: Use your calculator to evaluate the following
to
three decimal places.
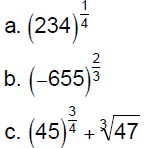
Answers Section 10.2
Example 1:
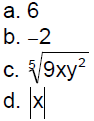
Example 2:
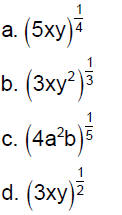
Example 3:
a. 64
b. 4
c. Not a real number
d. −8
Example 4:
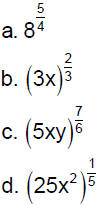
Example 5:
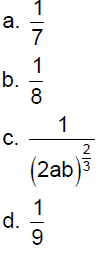
Example 6:
Example 7:
Example 8:
a. x = 55 kg., f(55)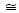 
1414 calories
Example 9:
a. 3.911
b. 75.421
c. 20.983