Math Test #1 Solutions

Solve the following equations:

(a) (3 + 2x)(1 - 3x) = (6x - 1)(1 - x),

(b) x(10x - 11) = 6

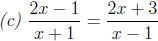
Solutions: (a) Expand both sides of the equation to obtain:

(3+2x)(1-3x) = (6x-1)(1-x) 3-9x+2x-6x² = 6x-6x²-1+x

or

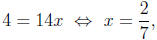
and so the solution written in set notation is

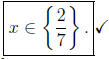
(b) Using the distributive property again we have

x(10x - 11) = 6 10x² - 11x = 6 10x² - 11x - 6 = 0:

Since the factorization method is not easy to apply here and also
the method of completing the square is still not very easy we apply
the quadratic formula a = 10, b = -11, c = -6:

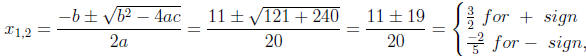
or Observe that 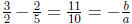and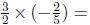
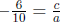which means the 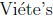relations are satisfied.

(c) Eliminating the denominators we have

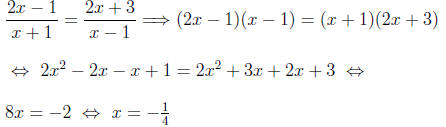
then after checking we see the only solution is

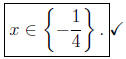
Find all real solutions of the radical equations:

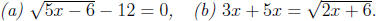
Solutions: (a) Squaring both sides, after isolating the radical ex-
pression, we get just an implication but good enough to see how the
solutions might look like:

or x = 30 and after checking we see that

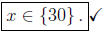
(b) Using the same method we get

32x² - x - 3 = 0.

Using the quadratic formula

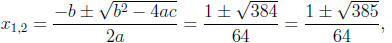
of which gives only one solution

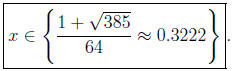
(a) A motorboat maintained a constant speed of 18 miles per hour
relative to the water in going 8 miles upstream and then returning.
The total time for the trip was 1 hours. Use this information to find
the speed of the current.

(b) If instead of knowing that it took 1 hours for the round trip
we know that it took 20 minutes more to go upstream than to go
downstream what would be the speed of the current in this case?

Solutions: (a) If we denote by x the speed of current in miles per
hour we get that the time going upstream is 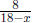(hours) and the
time going downstream is 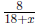(hours). Hence because it takes 1
hour round trip we obtain the equation:

Solving this for x by adding to the same common denominator we
get:

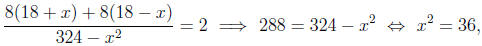
which gives .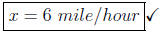

(b) Since 20 minutes is 1/3 of an hour. In this case the equation
becomes

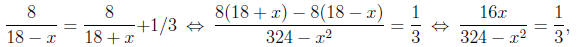
which implies



This gives only the solution .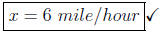

Solve the following inequalities and write your answers in in-
terval notation:

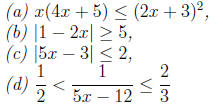
Solutions: (a) The inequality is equivalent to

which means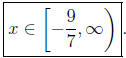

(b) The inequality becomes

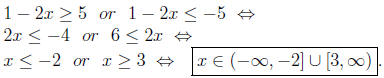
(c) In this case we have similarly

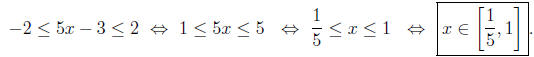
(d) Since we are dealing with positive numbers the inequality is
equivalent to

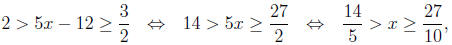
which gives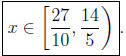

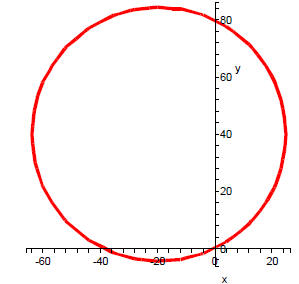
(a) Find the center and the radius of the circle of equation

x² + y² + 39x - 80y = 0.

(b) What are the coordinates of the x-intercepts ?

Solutions: (a) The equation can be written after completing the
squares

or

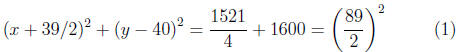
which gives the center 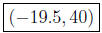and radius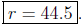

(b) Making y = 0 in x²+y²+39x-80y = 0 we obtain x²+39x = 0
which leads to two points of intersection: 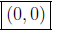and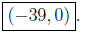
Similarly for the y-intercept we get: 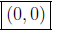and 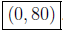. The graph
of this circle is included below:

Find the equation of the line in slope intercept form containing
the point of coordinates (3,-2) and parallel to the line of equation
7x + 5y - 3 = 0.

Solutions: The slope of the given line is m = 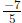. Hence the
equation of the line we are looking for is y - (-2) = (-7/5)(x - 3)
or .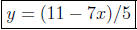

Find the domain of the following functions

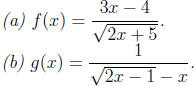
Solutions: (a) We need to impose the condition:



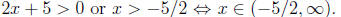
Hence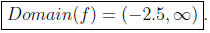

(b) In this case we have:

1. 2x - 1 ≥ 0 or 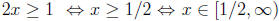and

2. or . If x ≥ 0, this is equivalent
to 2x - 1 = x² or 0 = x² - 2x + 1 (completing the square)
(x - 1)² = 0. We have only one non-negative solution: x = 1.
Therefore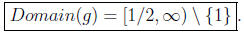

THE END