INTEGRATED ARITHMETIC AND ALGEBRA
| COURSE DESCRIPTION: (750 characters, maximum) A course designed to satisfy the requirements of both MAT 0012 and MAT 0024 in one semester. Topics to be studied include arithmetic with whole numbers, integers & rational numbers, linear equations and inequalities in one variable, factoring, laws of exponents, and basic linear graphing. Problem solving involving real-life scenarios is an integral part of this course. This course will teach students to understand and communicate concepts of algebra in the language of mathematics, both orally and written. This course helps prepare students for college-level mathematics and math-based courses. It is nontransferable. Due to the nature of this course, calculators are not permitted. To pass the course, students must pass a mandatory Florida State Examination. |
UNIT TITLES
1. Whole Numbers: Operations and Applications
2. Integers: Operations and Applications
3. Fractions & Mixed Numbers: Operations and Applications
4. Decimal Numbers: Operations and Applications
5. Linear Equations and Inequalities in One Variable
6. Ratios, Rates, Proportions, and Percents
7. Rules of Integer Exponents
8. Polynomial Expressions, Quadratic Expressions, & Quadratic Equations
9. Rational Expressions
10. Radical Expressions
11. Geometric Calculations, Charts and Graphs
12. Measurements and Unit Analysis
13. The Rectangular Coordinate System
14.
Lines, Slope, & Graphical Solutions to Systems of Linear Equations
15.
Sets (Optional)
EVALUATION:
Please provide a brief description (250 characters maximum) that details how
students will be evaluated on the course outcomes.
Written Quizzes/Examinations.
Cumulative Final Examination.
*** Complete the following only if course is seeking general education status ***
GENERAL EDUCATION Competencies and Skills *:
Please highlight in green font all Competencies/Skills from the list below that
apply to this course. In the box to the right of the
Competency/Skill, enter all specific learning outcome numbers (i.e. 1.1, 2.7,
5.12) that apply.
| 1. Read with critical comprehension | |
| 2. Speak and listen effectively | |
| 3. Write clearly and coherently | |
| 4. Think creatively, logically,
critically, and reflectively (analyze, synthesize, apply, and evaluate) |
|
| 5. Demonstrate and apply literacy in
its various forms: (highlight in green ALL that apply) ( 1. technological, 2. informational, 3. mathematical, 4. scientific, 5. cultural, 6. historical, 7. aesthetic and/or 8. environmental ) |
|
| 6. Apply problem solving techniques to real-world experiences | |
| 7. Apply methods of scientific inquiry | |
| 8. Demonstrate an understanding of
the physical and biological environment and how it is impacted by human beings |
|
| 9. Demonstrate an understanding of and appreciation for human diversities and commonalities | |
| 10. Collaborate with others to achieve common goals. | |
| 11. Research, synthesize and produce original work | |
| 12. Practice ethical behavior | |
| 13. Demonstrate self-direction and self motivation | |
| 14. Assume responsibility for and understand the impact of personal behaviors on self and society | |
| 15.Contribute to the welfare of the community |
* General Education Competencies and Skills endorsed by ’05-’06 General Education Task Force
Common Course Number: MAT 0020
Unit 1 Whole Numbers: Operations and Applications
General Outcome:
1.0 The student shall be able to perform operations involving whole numbers and solve appropriate word problems without the aid of a calculator.
Specific Measurable Learning Outcomes:
Upon successful completion of this unit, the student shall be able to:
1.1 Graph whole numbers on a number line.
1.2 Identify the place values of each digit of a whole number.
1.3 Write whole numbers using words, and vice versa.
1.4 Round whole numbers to a given place value.
1.5 Determine which of two whole numbers is greater using inequality symbols.
1.6 Identify the properties and words/phrases associated with the operations of addition, subtraction, multiplication, division, and exponentiation of whole numbers (e.g. sum, quotient, less than, a multiple of, cubed).
1.7 Translate word phrases into mathematical expressions, and vice versa (e.g. five less than twice a number = 2n – 5).
1.8 Apply “shortcut rules” for divisibility by 2, 3, 4, 5, 6, 9, and 10.
1.9 Add, subtract, multiply, divide, and exponentiate whole numbers.
1.10 Estimate sums, differences, products, and quotients of whole numbers by rounding.
1.11 Simplify numerical expressions using the order of operations.
1.12 Define the terms “prime number” and “composite number.”
1.13 Explain why the number one (1) is not prime.
1.14 Determine whether a whole number is prime, composite, or neither.
1.15 Determine all factors of whole numbers.
1.16 Determine the prime factorization of whole numbers.
1.17 Solve appropriate arithmetic word problems using operations on whole numbers.
Unit 2 Integers: Operations and Applications
General Outcome:
2.0 The student shall be able to perform operations involving integers and solve appropriate word problems without the aid of a calculator.
Specific Measurable Learning Outcomes:
Upon successful completion of this unit, the student shall be able to:
2.1 Graph integers on a number line.
2.2 Define the term “absolute value” with respect to distance.
2.3 Define the term “nonnegative,” and be able to distinguish it from the term “positive.”
2.4 Evaluate the absolute value of numbers and of
numerical expressions 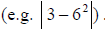
2.5 Determine which of two integers is greater using inequality symbols.
2.6 Identify the properties and words/phrases associated with the operations of addition, subtraction, multiplication, division, and exponentiation of integers.
2.7 Translate word phrases into mathematical expressions, and vice versa.
2.8 Add, subtract, multiply, divide, and exponentiate integers.
2.9 Simplify numerical expressions using the order of operations.
2.10 Solve appropriate arithmetic word problems using operations on integers.
Unit 3 Fractions & Mixed Numbers: Operations and Applications
General Outcome:
3.0 The student shall be able to perform operations involving fractions and mixed numbers and solve appropriate word problems without the aid of a calculator.
Specific Measurable Learning Outcomes:
Upon successful completion of this unit, the student shall be able to:
3.1 Identify a fraction’s numerator and denominator, and explain what each represents.
3.2 Represent fractions pictorially, and determine the fraction represented by a figure (e.g. the portion of a shaded figure).
3.3 Determine when a fraction is undefined.
3.4 Define the terms “proper fraction,” “improper fraction,” and “mixed number.”
3.5 Identify fractions as proper or improper.
3.6 Write improper fractions as mixed numbers, and vice versa.
3.7 Graph fractions and mixed numbers on a number line.
3.8 Determine equivalent representations of integers, fractions and mixed numbers.
3.9 Reduce fractions and mixed numbers to lowest terms.
3.10 Determine the least common denominator of two fractions.
3.11 Identify which of two fractions/mixed numbers is greater using inequality symbols.
3.12 Determine the reciprocal of integers, fractions, and mixed numbers.
3.13 Identify the properties and words/phrases associated with the operations of addition, subtraction, multiplication, division, and exponentiation of fractions and mixed numbers.
3.14 Translate word phrases into mathematical expressions, and vice versa.
3.15 Add, subtract, multiply, divide, and exponentiate fractions and mixed numbers.
3.16 Simplify complex fractions.
3.17 Simplify numerical expressions using the order of operations.
3.18 Solve appropriate arithmetic word problems using operations on fractions and mixed numbers.
Unit 4 Decimal Numbers: Operations and Applications
General Outcome:
4.0 The student shall be able to perform operations involving decimal numbers and solve appropriate word problems without the aid of a calculator.
Specific Measurable Learning Outcomes:
Upon successful completion of this unit, the student shall be able to:
4.1 Graph decimal numbers on a number line.
4.2 Identify the place values of each digit of a decimal number.
4.3 Round a decimal number to a given place value.
4.4 Rewrite terminating decimal numbers as a fraction or a mixed number, and vice versa.
4.5 Rewrite appropriate fractions as repeating decimals.
4.6 Define the terms “rational number,” “irrational number,” and “real number.”
4.7 Identify decimal numbers and square roots as rational or irrational.
4.8 Identify which of two decimal numbers is greater using inequality symbols.
4.9 Identify the properties and words/phrases associated with the operations of
addition, subtraction, multiplication, division, and exponentiation of decimal
numbers.
4.10 Translate word phrases into mathematical expressions, and vice versa.
4.11 Add, subtract, multiply, divide, and exponentiate decimal numbers.
4.12 Estimate sums, differences, products, and quotients of decimal numbers by rounding.
4.13 Add, subtract, multiply, divide, and exponentiate rational numbers
presented in any mixture of formats, and simplify numerical expressions using
the order of operations 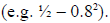
4.14 Evaluate the mean, median, mode, and range of lists of real numbers.
4.15 Solve appropriate arithmetic word problems using operations on real numbers.


